Weighted Marginal Utility
When money is limited and prices vary, every purchase becomes a trade-off. So how do smart consumers get the most satisfaction from their spending?
It all comes down to a key concept in economics: weighted marginal utility. It's the idea that helps us make better, more efficient choices about what to buy.
Marginal Utility, Explained
Let’s start with the basics. Marginal utility is the extra satisfaction you get from consuming one more unit of a good.
But here’s the catch: the more you consume, the less satisfaction each additional unit tends to bring. Economists call this the law of diminishing marginal utility.
Think about dessert: the first slice of cake is amazing. By the fourth, you might regret everything.
Real-world example. Let’s say eating one apple gives you 10 units of satisfaction (utility). The second apple gives 7, the third only 4. The more you eat, the less rewarding it gets.
This is why rational consumers aim to equalize the marginal utility of the last unit consumed across all goods.
Doing so spreads your income in the most satisfying way possible - maximizing your overall happiness from consumption.
But there’s another piece to the puzzle: price.
Same Prices? Compare Marginal Utilities
If all goods cost the same, life’s easy: you get the most value when the marginal utility is equal across the board.
$$ MU_A = MU_B = MU_C $$
So it’s not about buying what you love the most overall - it's about what gives you the most satisfaction per euro (or dollar) spent.
Real-world example. Imagine apples, pears, and bananas all cost €1. You’ll get the best value by buying a mix where the last apple, pear, and banana each give you the same level of utility. If that happens when you buy 5 apples, 3 pears, and 2 bananas, then that’s your sweet spot.
It’s like managing your free time. Whether you're exercising, hanging out with friends, or relaxing at home, no single activity should leave you wishing you’d done something else. If it does, your time wasn’t spent wisely. The same logic applies to spending money.
Different Prices? Time to Weigh Your Choices
When prices vary, you need to be more strategic. What matters isn’t just the utility of a good - it’s the utility you get per euro.
That leads us to the golden rule of smart consumption:
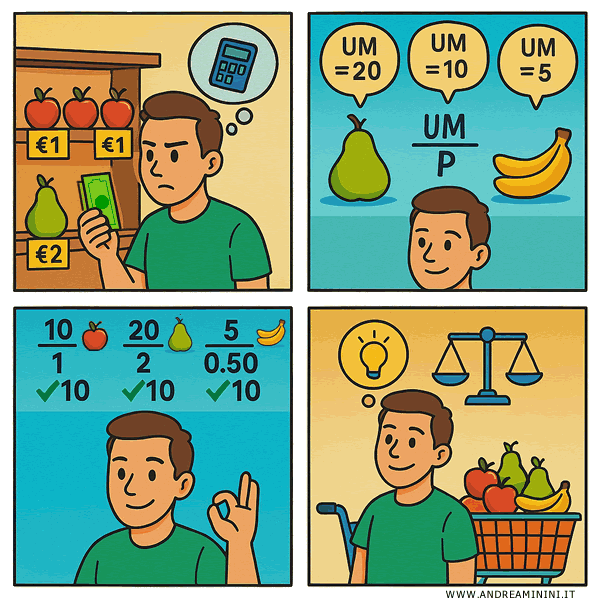
\[ \frac{MU_A}{P_A} = \frac{MU_B}{P_B} = \dots = \frac{MU_n}{P_n} \]
Translation? The last euro you spend on any good should bring you the same amount of satisfaction.
Real-world example. Say an apple costs €1, a pear €2, and a banana €0.50. You can’t just compare how much you like them - you need to weigh their marginal utility against the price.
| Good | Price (€) | Marginal Utility | Utility per Euro |
|---|---|---|---|
| Apple | 1.00 | 10 | 10 / 1 = 10 |
| Pear | 2.00 | 20 | 20 / 2 = 10 |
| Banana | 0.50 | 5 | 5 / 0.5 = 10 |
Here’s what’s happening: each of those purchases gives you exactly 10 units of utility per euro. That means you’ve nailed your spending choices - every euro is working equally hard. When that balance is achieved, economists call it consumer equilibrium.
So, What’s the Takeaway?
Weighted marginal utility helps you know when to keep buying a product - and when to switch gears.
If the next unit of something gives you less satisfaction per euro than another product, it’s time to pivot.
The smart consumer doesn’t necessarily spend more - they spend better.
In the end, maximizing satisfaction isn’t about getting more stuff - it’s about getting the most value for your money.
That’s how you reach consumer equilibrium. Or at least, that's the theory. In real life, temptation has a way of rewriting the rules...
